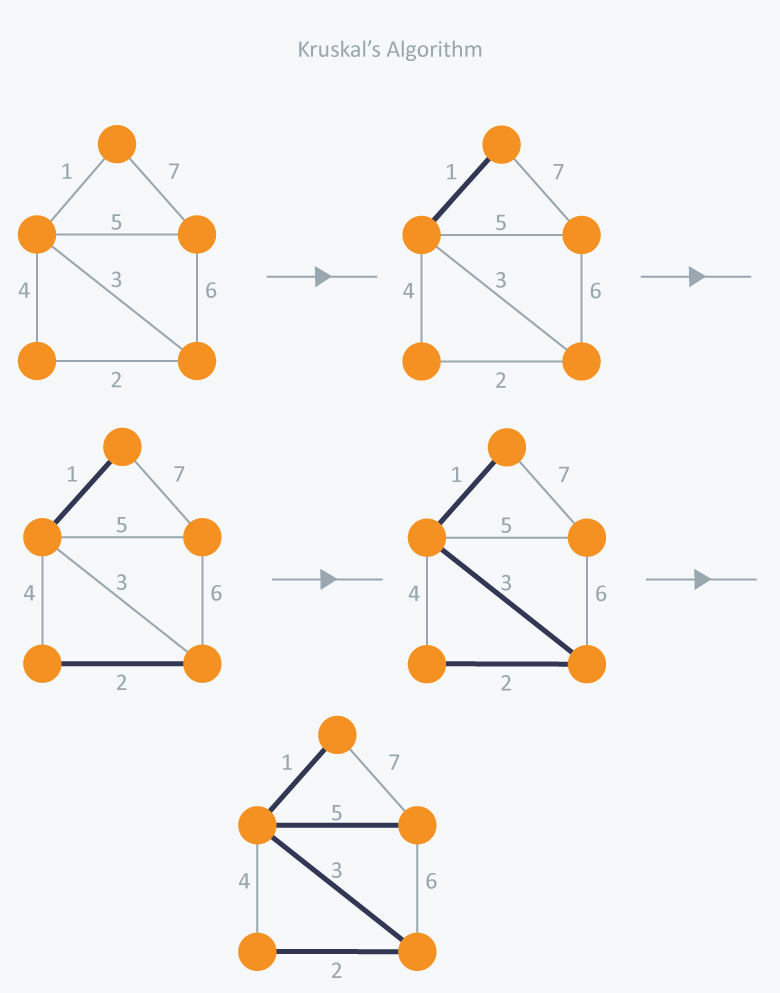
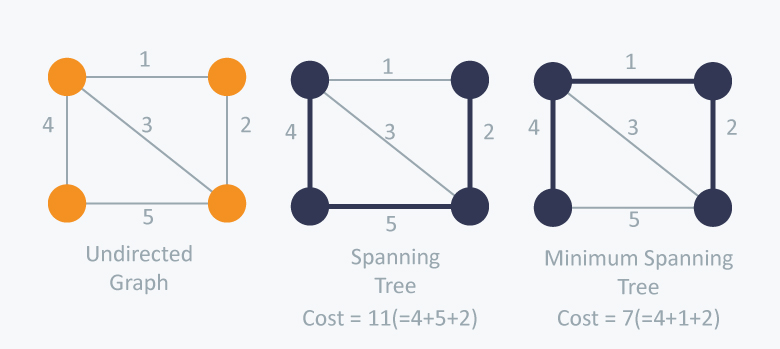
X.25 protocol

X.25

X.25 is a protocol suite defined by ITU-T for packet switched communications over WAN (Wide Area Network). It was originally designed for use in the 1970s and became very popular in 1980s. Presently, it is used for networks for ATMs and credit card verification. It allows multiple logical channels to use the same physical line. It also permits data exchange between terminals with different communication speeds.

X.25 has three protocol layers

•  Physical Layer: It lays out the physical, electrical and functional characteristics that interface between the computer terminal and the link to the packet switched node. X.21 physical implementer is commonly used for the linking.
• Data Link Layer: It comprises the link access procedures for exchanging data over the link. Here, control information for transmission over the link is attached to the packets from the packet layer to form the LAPB frame (Link Access Procedure Balanced). This service ensures a bit-oriented, error-free, and ordered delivery of frames.
• Packet Layer: This layer defines the format of data packets and the procedures for control and transmission of the data packets. It provides external virtual circuit service. Virtual circuits may be of two types: virtual call and permanent virtual circuit. The virtual call is established dynamically when needed through call set up procedure, and the circuit is relinquished through call clearing procedure. Permanent virtual circuit, on the other hand, is fixed and network assigned.

Characteristics of X.25

In addition to the characteristics of the packet switched network, X.25 has the following characteristics:

1.      Multiple logical channels can be set on a single physical line

2.      Terminals of different communication speeds can communicate

3.      The procedure for transmission controls can be changed.

The three layers of X.25 interface are as shown in Fig.(a).

• At the physical level X.21 physical interface is being used which is defined for circuit switched data network. At the data link level, X.25 specifies the link access procedure-B (LAP-B) protocol which is a subset of HDLC protocol.

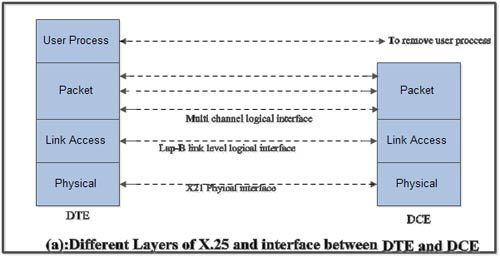
 At the network level (3^rd level), X.25 defines a protocol for an access to packet data subnetwork.

• This protocol defines the format, content and procedures for exchange of control and data transfer packets. The packet layer provides an external virtual circuit service.

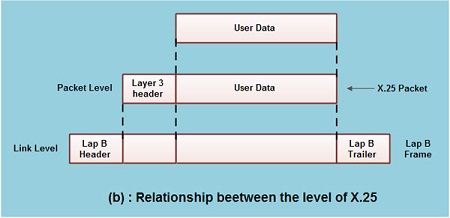
• Fig.(b) shows the relationship between the levels of x'25. User data is passed down to X.25 level 3.

• This data then appends the control information as a header to form a packet. This control .information is then used in the operation of the protocol.

 The entire X.25 packet formed at the packet level is then passed down to the second layer i.e. the data link layer.

The control information is appended at the front and back of the packet forming a LAP-B frame. The control information in LAP-B frame is needed for the operation of the LAP-B protocol.

• This frame is then passed to the physical layer for transmission.

Virtual Circuit Service

• With the X25 packet layer, data are transmitted in packets over external virtual circuits, The virtual circuit service of X25 provides for two types of virtual circuits,

• The virtual circuit service of X25 provides for two types of virtual circuits i.e. "virtual call" and "permanent virtual circuit".

• A virtual call is a dynamically established virtual circuit using a call set up and call clearing procedure.

• A permanent virtual circuit is a fixed, network assigned virtual circuit. Data transfer takes place as with virtual calls, but no call set up or clearing is required.

Prim’s Algorithm( Minimum spanning tree)

Prim’s Algorithm

Prim’s Algorithm also use Greedy approach to find the minimum spanning tree. In Prim’s Algorithm we grow the spanning tree from a starting position. Unlike an edge in Kruskal's, we add vertex to the growing spanning tree in Prim's.

Algorithm Steps:

• Maintain two disjoint sets of vertices. One containing vertices that are in the growing spanning tree and other that are not in the growing spanning tree.
• Select the cheapest vertex that is connected to the growing spanning tree and is not in the growing spanning tree and add it into the growing spanning tree. This can be done using Priority Queues. Insert the vertices, that are connected to growing spanning tree, into the Priority Queue.
• Check for cycles. To do that, mark the nodes which have been already selected and insert only those nodes in the Priority Queue that are not marked.

Consider the example below:

In Prim’s Algorithm, we will start with an arbitrary node (it doesn’t matter which one) and mark it. In each iteration we will mark a new vertex that is adjacent to the one that we have already marked. As a greedy algorithm, Prim’s algorithm will select the cheapest edge and mark the vertex. So we will simply choose the edge with weight 1. In the next iteration we have three options, edges with weight 2, 3 and 4. So, we will select the edge with weight 2 and mark the vertex. Now again we have three options, edges with weight 3, 4 and 5. But we can’t choose edge with weight 3 as it is creating a cycle. So we will select the edge with weight 4 and we end up with the minimum spanning tree of total cost 7 ( = 1 + 2 +4).

Time Complexity:
The time complexity of the Prim’s Algorithm is $O((V+E)logV)$ because each vertex is inserted in the priority queue only once and insertion in priority queue take logarithmic time.

Kruskal’s Algorithm( Minimum Spanning Tree)

Kruskal’s Algorithm

Kruskal’s Algorithm builds the spanning tree by adding edges one by one into a growing spanning tree. Kruskal's algorithm follows greedy approach as in each iteration it finds an edge which has least weight and add it to the growing spanning tree.

Algorithm Steps:

• Sort the graph edges with respect to their weights.
• Start adding edges to the MST from the edge with the smallest weight until the edge of the largest weight.
• Only add edges which doesn't form a cycle , edges which connect only disconnected components.

So now the question is how to check if $2$ vertices are connected or not ?

This could be done using DFS which starts from the first vertex, then check if the second vertex is visited or not. But DFS will make time complexity large as it has an order of $O(V+E)$ where $V$ is the number of vertices, $E$ is the number of edges. So the best solution is "Disjoint Sets":
Disjoint sets are sets whose intersection is the empty set so it means that they don't have any element in common.

Consider following example:

In Kruskal’s algorithm, at each iteration we will select the edge with the lowest weight. So, we will start with the lowest weighted edge first i.e., the edges with weight 1. After that we will select the second lowest weighted edge i.e., edge with weight 2. Notice these two edges are totally disjoint. Now, the next edge will be the third lowest weighted edge i.e., edge with weight 3, which connects the two disjoint pieces of the graph. Now, we are not allowed to pick the edge with weight 4, that will create a cycle and we can’t have any cycles. So we will select the fifth lowest weighted edge i.e., edge with weight 5. Now the other two edges will create cycles so we will ignore them. In the end, we end up with a minimum spanning tree with total cost 11 ( = 1 + 2 + 3 + 5).

Time Complexity:
In Kruskal’s algorithm, most time consuming operation is sorting because the total complexity of the Disjoint-Set operations will be $O(ElogV)$, which is the overall Time Complexity of the algorithm.

Minimum Spanning Tree

What is a Spanning Tree?

Given an undirected and connected graph $G=(V,E)$, a spanning tree of the graph $G$ is a tree that spans $G$ (that is, it includes every vertex of $G$) and is a subgraph of $G$ (every edge in the tree belongs to $G$)

What is a Minimum Spanning Tree?

The cost of the spanning tree is the sum of the weights of all the edges in the tree. There can be many spanning trees. Minimum spanning tree is the spanning tree where the cost is minimum among all the spanning trees. There also can be many minimum spanning trees.

Minimum spanning tree has direct application in the design of networks. It is used in algorithms approximating the travelling salesman problem, multi-terminal minimum cut problem and minimum-cost weighted perfect matching. Other practical applications are:

1. Cluster Analysis
2. Handwriting recognition
3. Image segmentation     

There are two famous algorithms for finding the Minimum Spanning Tree:

Kruskal’s Algorithm

Kruskal’s Algorithm builds the spanning tree by adding edges one by one into a growing spanning tree. Kruskal's algorithm follows greedy approach as in each iteration it finds an edge which has least weight and add it to the growing spanning tree.

Prim’s Algorithm

Prim’s Algorithm also use Greedy approach to find the minimum spanning tree. In Prim’s Algorithm we grow the spanning tree from a starting position. Unlike an edge in Kruskal's, we add vertex to the growing spanning tree in Prim's.